Skip to contents

Plot Interaction Effect Using the Johnson-Neyman Technique

plot_jn.Rd

This function plots the simple slopes of an interaction effect across different values of a moderator variable using the Johnson-Neyman technique. It identifies regions where the effect of the predictor on the outcome is statistically significant.

Usage

plot_jn(
  x,
  z,
  y,
  model,
  min_z = -3,
  max_z = 3,
  sig.level = 0.05,
  alpha = 0.2,
  detail = 1000,
  sd.line = 2,
  standardized = FALSE,
  xz = NULL,
  greyscale = FALSE,
  ...
)

Arguments

x

The name of the predictor variable (as a character string).

z

The name of the moderator variable (as a character string).

y

The name of the outcome variable (as a character string).

model

A fitted model object of class modsem_da, modsem_mplus, modsem_pi, or lavaan.

min_z

The minimum value of the moderator variable z to be used in the plot (default is -3). It is relative to the mean of z.

max_z

The maximum value of the moderator variable z to be used in the plot (default is 3). It is relative to the mean of z.

sig.level

The alpha-criterion for the confidence intervals (default is 0.05).

alpha

alpha setting used in ggplot (i.e., the opposite of opacity)

detail

The number of generated data points to use for the plot (default is 1000). You can increase this value for smoother plots.

sd.line

A thick black line showing +/- sd.line * sd(z). NOTE: This line will be truncated by min_z and max_z if the sd.line falls outside of [min_z, max_z].

standardized

Should coefficients be standardized beforehand?

xz

The name of the interaction term. If not specified, it will be created using x and z.

greyscale

Logical. If TRUE the plot is plotted in greyscale.

...

Additional arguments (currently not used).

Value

A ggplot object showing the interaction plot with regions of significance.

Details

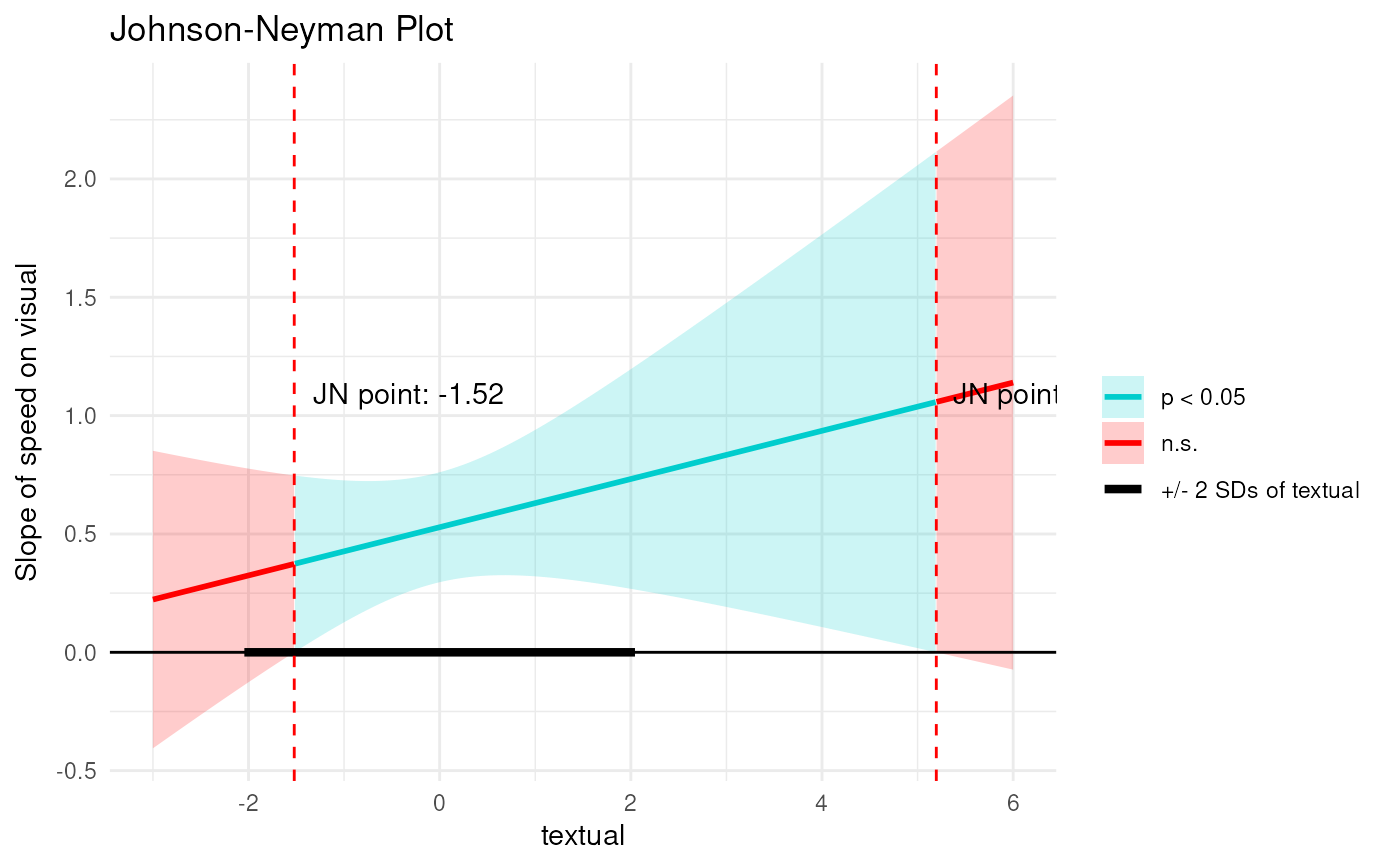
The function calculates the simple slopes of the predictor variable x on the outcome variable y at different levels of the moderator variable z. It uses the Johnson-Neyman technique to identify the regions of z where the effect of x on y is statistically significant.

It extracts the necessary coefficients and variance-covariance information from the fitted model object. The function then computes the critical t-value and solves the quadratic equation derived from the t-statistic equation to find the Johnson-Neyman points.

The plot displays:

  • The estimated simple slopes across the range of z.

  • Confidence intervals around the slopes.

  • Regions where the effect is significant (shaded areas).

  • Vertical dashed lines indicating the Johnson-Neyman points.

  • Text annotations providing the exact values of the Johnson-Neyman points.

Examples

# \dontrun{
library(modsem)

m1 <-  '
  visual  =~ x1 + x2 + x3
  textual =~ x4 + x5 + x6
  speed   =~ x7 + x8 + x9

  visual ~ speed + textual + speed:textual
'

est <- modsem(m1, data = lavaan::HolzingerSwineford1939, method = "ca")
plot_jn(x = "speed", z = "textual", y = "visual", model = est, max_z = 6)

# }